Prandtl Number – Mechanical Engineering

The Prandtl number (Pr) or Prandtl group is a dimensionless number, defined as the ratio of momentum diffusivity to thermal diffusivity. That is, the Prandtl number is given as:

\mathrm{Pr} = \frac{\nu}{\alpha} = \frac{\mbox{viscous diffusion rate}}{\mbox{thermal diffusion rate}} = \frac{\mu / \rho}{k / c_p \rho} = \frac{c_p \mu}{k}

where:

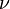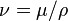$\nu$ : momentum diffusivity (kinematic viscosity), $\nu = \mu/\rho$ , (SI units: m²/s)
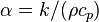$\alpha$ : thermal diffusivity, $\alpha = k/(\rho c_p)$ , (SI units: m²/s)
$\mu$ : dynamic viscosity, (SI units: Pa s = N s/m²)
$k$ : thermal conductivity, (SI units: W/m-K)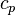
$c_p$ : specific heat, (SI units: J/kg-K)
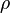$\rho$ : density, (SI units: kg/m³)

Small values of the Prandtl number, $Pr << 1$ , means the thermal diffusivity dominates. Whereas with large values, $Pr >> 1$ , the momentum diffusivity dominates the behavior. For example, for liquid mercury the heat conduction is more significant compared to convection, so thermal diffusivity is dominant. However, for engine oil, convection is very effective in transferring energy from an area in comparison to pure conduction, so momentum diffusivity is dominant.

In heat transfer problems, the Prandtl number controls the relative thickness of the momentum and thermal boundary layers. When $Pr$ is small, it means that the heat diffuses quickly compared to the velocity (momentum). This means that for liquid metals the thickness of the thermal boundary layer is much bigger than the velocity boundary layer.